Information on the author

Yuri V. Teplinsky

Anatolii Mykhailovych Samoilenko (on his 80th birthday): A. A. Boichuk, W. L. Kułyk, I. O. Parasyuk, M. O. Perestyuk, R. I. Petryshyn, G. P. Pelyukh, M. Rontó, O. M. Stanzhitskyi, Y. V. Teplinsky, V. I. Tkachenko, S. I. Trofimchuk, and S. M. Chuiko; Volume 21, No.1, pp. 3-5; Link PDF
Of blessed memory of a prominent mathematician, Anatolii Mykhailovych Samoilenko (02.01.1938 – 04.12.2020): A. A. Boichuk, W. L. Kułyk, I. O. Lukovsky, V. L. Makarov, I. O. Parasyuk, G. P. Pelyukh, M. O. Perestyuk, R. I. Petryshyn, A. K. Prykarpatski, A. N. Ronto, M. Rontó, V. H. Samoilenko, O. M. Stanzhitskyi, Y. V. Teplinsky, O. M. Tymokha, V. I. Tkachenko, S. I. Trofimchuk, and S. M. Chuiko; Volume 26, No.1, pp. 3-5; Link PDF
Oleksandr Andriyovych Boichuk (on his 65th birthday): A. M. Samoilenko, I. T. Kiguradze, I. O. Lukovsky, V. L. Makarov, M. O. Perestyuk, O. M. Sharkovsky, J. Diblík, V. F. Zhuravlev, M. Medveď, I. O. Parasyuk, N. H. Rozov, O. M. Stanzhitskyi, Y. V. Teplinsky, V. I. Tkachenko, and M. Fečkan; Volume 18, No.2, pp. 147-148; Link PDF
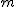About $C^\varrho$ -smootness of an invariant torus of a countable system of difference equations defined on an $m$ -dimensional torus: Y. V. Teplinsky and N. A. Marchuk; Volume 5, No.2, pp. 251-265; Link PDF
On count-point boundary value problems for countable systems of ordinary differential equations: Y. V. Teplinsky and V. A. Nedokis; Volume 2, No.2, pp. 252-266; Link PDF
On existence of infinite-dimensional invariant tori for nonlinear countable systems of differential-difference equations: A. M. Samoilenko, Y. V. Teplinsky, and K. V. Pasyuk; Volume 13, No.2, pp. 253-271; Link PDF
On Frechet differentiability of invariant torus for nonlinear countable system of difference equations defined on an infinite-dimensional torus and containing deviations of the discrete argument: Y. V. Teplinsky and N. A. Marchuk; Volume 6, No.2, pp. 260-278; Link PDF
A construction of an infinite dimensional invariant torus for a countable system of linear differential-difference equations via the method of reduction in the angular variable: A. M. Samoilenko, Y. V. Teplinsky, and K. V. Pasyuk; Volume 14, No.2, pp. 267-280; Link PDF
Oleksandr Andriiovych Boichuk (on 60-th anniversary of his birthday): A. M. Samoilenko, M. O. Perestyuk, J. Diblík, M. Medveď, I. O. Parasyuk, R. I. Petryshyn, O. M. Stanzhitskyi, Y. V. Teplinsky, V. I. Tkachenko, M. Fečkan, and S. M. Chuiko; Volume 13, No.2, pp. 287-288; Link PDF
On existence of invariant tori for countable systems of differential-difference equations defined on infinite-dimensional tori: A. M. Samoilenko, Y. V. Teplinsky, and K. V. Pasyuk; Volume 12, No.3, pp. 347-367; Link PDF
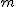
On existence of a smooth bounded semiinvariant manifold for a degenerate nonlinear system of difference equations in the space $\mathfrak{m}$: A. M. Samoilenko, Y. V. Teplinsky, and I. V. Semenyshyna; Volume 6, No.3, pp. 378-400; Link PDF
Boundary-value problems for differential equations not solved with respect to the derivative, in the space of bounded number sequences: A. M. Samoilenko, Y. V. Teplinsky, and V. A. Nedokis; Volume 10, No.3, pp. 391-415; Link PDF
On finding periodic solutions of second order difference equations in a Banach spase: Y. V. Teplinsky and I. V. Semenyshyna; Volume 4, No.3, pp. 405-421; Link PDF
On periodic solutions of difference equations in infinite-dimensional spaces: Y. V. Teplinsky and I. V. Semenyshyna; Volume 3, No.3, pp. 414-430; Link
International scientific conference "Differential equations and their applications": A. M. Samoilenko, M. O. Perestyuk, V. H. Samoilenko, I. M. Konet, and Y. V. Teplinsky; Volume 20, No.3, pp. 431-432; Link PDF
On a nonlinear boundary-value problem on the half-line for ordinary differential equations in the space of bounded number sequences with a countable set of boundary moments: Y. V. Teplinsky and V. A. Nedokis; Volume 6, No.4, pp. 530-549; Link PDF
On the invariant tori of quasilinear countable systems of differential equations defined on infinite-dimensional tori: Y. V. Teplinsky; Volume 23, No.4, pp. 553-564; Link PDF